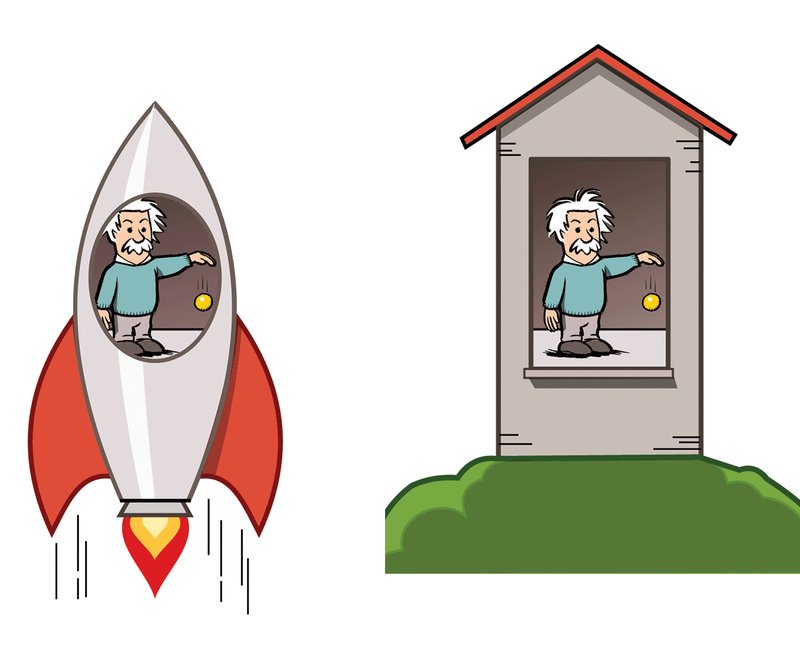
Einstein's principle of equivalence

The example of the spaceship offers much deeper information. If during the trip you had an extremely precise clock, you might observe that the time T on the ship runs differently according to the "height" S with respect to the "ground floor" (base of the spaceship).

In particular, the more we rise in height, the more the time runs quickly according to the equation:

The reason is simple but indeed counterintuitive. Our intuition would tell us that in order to maintain a constant distance between two objects accelerated in a certain direction (in this case, two components with different "height" in the spaceship) it is necessary for them to have the same acceleration. But intuition in this case deceives us and has also confused several researchers over the years, leading to the so-called Bell's spaceship paradox. Actually, there is no paradox: the point is that relativistic effects are infinitesimal at the scales to which we are accustomed to, and are able to perceive, so relying on our intuition is simply wrong.

In general, if we take into account as a reference system the base of the spaceship and assume it is subject to a constant acceleration A, we can calculate the acceleration α(S) at distance (height) S, which results:

In order to maintain the same distance from the base (ground floor), the components of the spaceship in the direction of the acceleration (height) must reduce their acceleration, according to the equation just shown. And it is precisely this reduction in the acceleration (with respect to the base) to reduce time dilation effects, thus making time running quickly. Counterintuitive, but true!

And there is an even more important aspect. According to the principle of equivalence formulated by Albert Einstein, which underpins the whole General Relativity (see Muñoz and Jones 2010), the simple equation that measures the time at different height on the spaceship is also an excellent estimate of the differences in time that you might measure at different altitudes, in any house (e.g. at different floors) or between the sea level and the mountains.

You understand well, the time upstairs flows faster, as well as the time in the mountains compared to the sea. Over 100 years after its formulation, the equivalence principle has been tested experimentally with the incredible precision of one part in a million billion!

Let's make a concrete calculation

By setting a height difference of S = 10 meters, or

light years, for each time interval spent by those who are at the ground floor, the corresponding time interval at the top is equal to:

The difference in time is relative, i.e. it does not depend on the unit of measurement chosen for time (purely conventional). I used the light year simply because more comfortable (we had already calculated A=1.03 as speed increase per light year in previous article).

This difference is impossible to detect with normal watches (they should have accuracy of one millionth of billionth of a second), let alone with our senses.

Thanks to the increased accuracy of atomic clocks, however, we have managed to confirm with extreme accuracy the validity of these relations for speeds of less than 10 meters per second (36 km per hour) and for differences in height of less than one meter, respectively (Chou et al. 2010).

More recently, we have even been able to measure time differences for heights at millimeter scale (Bothwell et al. 2022).

Unconsciously, whenever we use geolocation on our smartphones and we see that works and it is precise, we are verifying the validity of Einstein's General Relativity. Clocks on satellites are both in motion and at a significant height from the ground, so corrections are made that take into account the different measurements of time on the ground and the satellite precisely applying Einstein's theory. In particular, the satellite clocks are adjusted lower in frequency by approximately half part in a billion, before sending them into orbit (see equation 36 in Ashby 2003).

Without this correction, time measurements would quickly get out of sync and lead to geolocation errors of kilometers in a single day (making, in fact, all geolocation infrastructure useless).